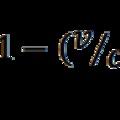The intelligent electron

Stage: The hydrogen atom

Actors: The physicist and the electron

Characters: The physicist represents the views of classical physics, while the electron can gain information only from the microscopic world.

. . . . . . . . . .

Physicist: Where are you going?

Electron: I go nowhere, I just exist.

Physicist: OK, but you have to circulate somehow on your orbit around the nucleus? Like the planets around the Sun!

Electron: Why should I circulate, when I am already on my orbit? Mostly I am away from the proton at a distance of the Bohr radius [1], but I am also at the site of the nucleus, and also far from there. I am everywhere a little bit.

 Physicist: It is impossible, one can be either here, or there, one cannot be at two places at the same time.  

Electron: Maybe you are not able, but I can do. I certainly should know it pretty well! Well, you asked me a question about circulation, but what does it mean?   

Physicist: Look the sky, you can see the sunrise and the motion of Sun from the east to the west until the sunset, and in the night you can follow the revolution of stars. Of course, we know the motion of stars and planets is virtual since the Earth rotates.  But if you take into account it, you can calculate the real orbits of the planets, which are described by the laws of Kepler [2].

Electron: But how you have the ability of seeing and how you are able the track the motion of planets? 

Physicist: That is thanks to the light and its tiny elements, the photons, which arrive in an enormous number, and by organizing all consecutive pictures you can draw the orbit of motion.   

Electron: But as concerning me, a sole photon can divert from my original orbit, or even can strip from the proton where I stayed before.  But until it does not happen, I stay in the same orbit. Until no photon turns into my way, I have no chance to observe consecutive events, there is no “before” or “after” in this state, so for me the concept of time does not exist.

Physicist (doubtful): But if the time does not exist, how you can speak about speed, acceleration, momentum and even kinetic energy?  

Electron: You are only partially right. Indeed we cannot speak about speed, acceleration or momentum unless they have the zero value [3]. I am bonded to the nucleus, any non-zero momentum would remove me from the nucleus, while the acceleration would force me emitting photons and losing my energy until the attraction power of nucleus would swallow me.  In spite of  missing speed, I do posses kinetic energy!

Physicist (still doubtful): But how is it possible if you do not have non-zero speed?

Electron: Because there is something that can replace time, my existence is not related to the time, but to the probability distribution in the space.  Naturally, you can hardly comprehend how the concept of probability distribution can define my existence, like for me the concept of time is not comprehensible since in my state no consecutive events can happen. What is common in our different worlds, the role of potential and kinetic energy, which can create the orbits by converting each other. While for you the orbits are developed in the course of time, for me it is expressed in the dimension of probability.

Physicist (strongly doubtful): It means that for the electrons no time, no motion, no displacement exist at all?  Only the probability distribution exists?

Electron:  By no means! What I have told you is valid only in the bonded, stationary states. By the way, one of my electron brothers is happen to be in a cyclotron [4], where he is forced into a circular orbit by the electromagnetic field. In this orbit he has acceleration and consequently continuous photon emission. In this way he perceives consecutive events giving him the chance to develop the concept of time. His motion can persist since in the course of radiation the loss of energy is compensated or even enhanced by the synchronized electromagnetic field.

Physicist : Let us return to your micro world. How the space distribution depends on the direction?  

Electron: But what do you mean on the word of direction?  As for me such a term does not exist. You can speak about direction because you perceive a lot of photons emitted by the surroundings and you can compare the information transferred by them. But what could I compare who cannot see a single photon?

Physicist (wondering): So you cannot perceive the space at all?

Electron: Oh no, I can, since the attraction power is changing with the distance from the proton. For example, inside the nucleus the attraction power is infinitely strong. I can feel this force by communicating with the proton mediated by the virtually emitted and absorbed photons.

Physicist (pensively):  There are two points that I cannot understand. You said just before that in the stationary state there is no photon emission, the other point concerns the question that why the proton cannot swallow you in the direct contact producing infinitely strong attraction?

Electron: Indeed none of the statements can be easily understood. Let us start with the emitted photons.  They constitute no observable photons since the electronic states are not modified. It is why one can call them as virtual. They are simultaneously emitted and absorbed without changing the stationary state of electrons.

Physicist (debating): But what is the meaning by speaking about something that cannot alter the state of electrons?

Electron: It has a meaning, since these photons build up the electromagnetic field. But this field is not static, it fluctuates around the equilibrium. This phenomenon is the vacuum polarization. 

Physicist (doubtful): I have to admit that I still cannot understand it!

Electron: Then I continue. A magnetic field can force us to precess [5] and we can choose between two senses of rotation yielding two energy levels. The difference of energy is proportional to the magnetic field and there is a constant, which tells us how large is the separation.  According to the relativistic quantum mechanics, this constant should be equal to 2, but the actual value is somewhat larger (2.0023…). This small deviation is caused by the vacuum polarization [6].

Physicist (pensively): I still cannot understand whether your explanation only refers to a mathematical procedure for reproducing a constant or these virtual photons do exist. But proceed further, why the infinitely strong attraction cannot swallow you in the nucleus?  

Electron (proudly):  Can I say, that is because we love freedom? The narrower is the cage where we are jammed the stronger we are bombing the walls! This phenomenon is called in science as the uncertainty relation [7]. In the narrow space you know precisely the position, but then the momentum becomes very uncertain.

Physicist (strongly doubtful): You said before that in bonded state no momentum exists!

Electron: Indeed, the average of momentum is zero and we cannot speak about the speed and momentum at a particular place inside the atom. But still exists something, what we can call as the standard deviation of momentum [8]. This standard deviation is increased in the narrow space, which is actually the square of momentum since its average value is zero. If you divide the square of momentum by the double of mass, you obtain the kinetic energy. Furthermore this kinetic energy increases in the vicinity of nucleus by a larger extent than the potential energy, which allows us for occupying an orbit that contains the site of nucleus without the risk to be swallowed

Physicist: When you speak about orbits in the atom, how you can differentiate them?

Electron: In a particular orbit I can occupy a certain position with a well defined probability, in certain orbits I can occupy even the site of nucleus, but in other orbits it is not possible.  There are separated regions of the orbits where the borders form forbidden zones for me.

Physicist (interrupting): Then how can you transfer through these zones?

Electron (reprimanding): I have already told you that I am everywhere, where I can be, I do not need transfer anywhere.

Physicist (wondering): So you are cut into pieces?

Electron (smiling): Definitely, not. I am an elementary particle, one and indivisible. You could not find in the whole universe any physical object with mass and charge, which have smaller mass than me. The existence of separated zones means that I can be simultaneously present in different regions of the space.  My orbit is organized by the probability distribution. But let us speak now about the orbits of planets. How can you distinguish them, how can you classify them?

Physicist: Of course, we can speak about not only the planets, but also about comets and artificial satellites circulating around the Sun or the Earth. The orbits can be characterized by their average distance from the Sun, as well as, the ratio of farthest and closest positions, this ration can be extremely large for the comets. The larger is the distance from the center the longer time is needed for a complete revolution, but what is interesting that this rule is the same for any objects if their mass is small compared to the Sun.   

Electron: No time dependence is defined in my atomic orbital, but there is a probability distribution, where the spatial dimension depends on the mass even though my mass is much smaller than that of the nucleus.  If my mass were twice larger, then my average distance from the nucleus would be twice smaller [9]. Why it is not so for the planets?

Physicist: What is matter, the ratio of the attraction power compared to the mass, and the gravitational force is proportional to the mass [10]

Electron: Now I realize why the situation is different, since in my case the attraction power of nucleus does not depend on the mass. I have, however, a further question: I guess planets can have only well defined distances from the Sun [11], and thus the respective energy can change  jump like.

Physicist: (not comprehending): Why you assume jumps, the planetary orbits can have any radius and any energy!

Electron (pensively): When I was still a free electron and approached the proton, the increasing attraction power accelerated me, which made me emit photons. All photons changed my angular momentum by the amount of the ℏ Planck constant. As a consequence when I landed on the atomic orbit my angular momentum was a multiple of ℏ.  When later I emitted or absorbed photons, always the angular momentum is changed by the same amount.  In the course of these events the number of maxima in the probability distribution was also changed, which is termed as the principal quantum number. Basically it determines the energy level of the orbits [12]. I wonder why it is not so for the planetary motion, perhaps it is caused by the different nature of gravitational force?  

Physicist (deeply thinking): It is a good question! We are still not sure if there are quanta in the gravitation. Since this interaction is rather weak, we can investigate this phenomenon only for objects possessing large mass, but in this case the angular momentum is also rather large making difficult to observe elementary jumps. But returning back to the microscopic objects, then the quantum nature of photons is responsible for the jump like changes in the energy?

Electron: You are right, the photons can select among the energy levels, where the allowed transitions can take place.

Additional topics in the blog, see: "Paradigmaváltás a fizikában"

1. The dimension of atomic orbits is determined by the Bohr radius, which can be given by the Planck constant as well as the mass and charge of the electron:
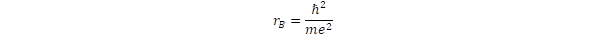
2. According to the second law of Kepler, the third power of average distance from the Sun is proportional to the square of the revolution period.
3. The momentum is defined by an imaginary operator, but any physical quantity should have real values. The latter condition is satisfied when the expectation value is computed by an integral extended to the whole size of the physical object. In the stationary states the momentum is imaginary in any point except the center; contrary to it the square is real. In bonded state the expectation value of momentum is zero, thus the square of momentum represents the standard deviation, which is proportional to the kinetic energy.
4. The cyclotron can accelerate the electron on a circular orbit.
5. The precession is a secondary rotation. An example is the precession of the Earth with the period 25 800 year, when the inclination of the rotation axis is turning around. The rotations can be either left- or right-handed.
6. The theory of quantum electrodynamics is the advanced level of quantum theory, when the interaction and the interacting particles are handled under a common principle. This theory assumes continuous emission and absorption of the virtual photons mediating the electromagnetic interactions. This phenomenon creates a fluctuation of the fields termed as the vacuum polarization.
7. According to the uncertainty relations one can never determine with infinite precision both the position and the momentum of a physical object. The product of errors should be at least as large as the Planck constant. Similarly the life time of any quantum mechanical state limits the precision of energy measurement.
8. See footnote 2.
9. See the first footnote, where the expression of the Bohr radius indicates the inverse proportionality with the mass.
10. The gravitational force g.m.M/R*² is counterbalanced by the centrifugal force  m.v*²/R .
11. The characteristic radius of the atomic orbits can be given as a product of n principal quantum number and the Bohr radius:  R_n = n.r_B
12. The energy of atomic orbits can be given by the characteristic radius: