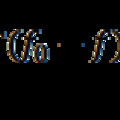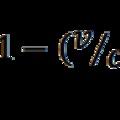Second part from Ind. J. Phys., 89, 389-396 (2015)

A screw model for quantum electrodynamics: From gravitation to quanta

A Rockenbauer*

Department of Physics, Budapest University of Technology and Economics and

MTA-BME Condensed Matter Research Group, Budafoki ut 8, 1111 Budapest, Hungary

Abstract

A screw model is developed for photons and fermions to offer a physical representation for the Feynman’s arrow scheme in quantum-electrodynamics. This model interprets intrinsic parameters of particles: spin, rest energy and magnetic moment by self-rotations with the speed of light forming either a helical (boson) or a spherical (fermion) screws. Due to the extreme Lorentz contraction the surface of screws is zero, while the radius remains finite (Compton radius). According to the general theory of relativity, the non-Euclidean geometry of space-time caused by the self-rotation of particles should produce an intrinsic force, which is analogous to the gravitation, but being 32-42 orders of magnitudes stronger, we denote it as strong gravitation. It is a self-stabilizing force for the intrinsic rotations, which is the source of spin and defines the Planck constant. The spherical screws of fermions are formed by double rotations, where the two rotations have right or left-handed chirality representing the particles and anti-particles. The double-rotation produces Coriolis force, where the sign is determined by the chirality and this force is the origin of electric charge. Parity violation in the beta-decay of neutrons is related to the symmetry of reflection for the self-motion of particles. The finite radius of self-rotation may resolve also divergences in the theory of quantum-electrodynamics.

Keywords: Feynman’s arrows; QED; self-motion of particles; strong gravitation

PACS (2010): 11.30.Er; 12.10.-g; 12.20.-m; 13.40.Em; 14.60.Cd

Corresponding author, e_mail: rockenbauer.antal@ttk.mta.hu

..............

Eq. (16) gives also the angular frequency w and the central radius R_c:

ω = 2m.c²/ħ     and     R_c = ħ/2m.c                                              (17)

There is a factor 2 in these expressions compared to the de Broglie frequency and the Compton wavelength [8], respectively, which is again related to the definition of the double rotation. Actually the de Broglie frequency is equal to the two-dimensional angular frequency .

Let us rewrite Eq. (16) in the form of the Kepler’s law:

ħ.c/2m = ω² R³_c                                                                       (18)

A comparison of Eq. (18) with Eq. (5) shows the ratio of strong to weak gravitation at the shell r = R_c :

F_sgr/F_gr = ħ.c/(2γ.m²)                                                                 (19)

In the case of electrons the ratio is 2.85x10⁴² , for nucleons it is around 10³⁶, and for the heaviest particles it is around 10³².  For elementary particles the frequency jump is also enormous: 10¹⁶-10²¹ at the borderline of shell r = R_c

According to the outlined screw model, the quantum nature of elementary processes is related to the strong gravitation, which separates rotating domains in the space and stabilizes both one- and two-dimensional rotations (bosons and fermions), where the particles have angular momenta ћ and ћ/2, respectively. All informations, obtained from the motions of fermions (e.g. the motion of electrons in the atoms) are mediated by absorptions and emissions of photons with the angular momentum of ħ. Thus all physical laws of micro-world are governed by the quantum unites of Planck constant. This principle is expressed in the most consequent manner in the theory of QED. For this reason our screw model can be considered as a conceptual support for the basic idea of quantum physics.

The fermions, like electrons, muons and tau particles have well defined mass, that is the frequency of the double-rotation cannot change continuously contrary to the case of photons. Why are only discrete frequencies of the self-rotation allowed for fermions? It might be possible that the particle generating mechanism, like the symmetry breaking of Higgs process [11], can produce particles only with well defined mass, or the two simultaneous rotations can resonate only at definite frequencies. Our screw model cannot answer this question. Similarly this problem is left open in the Standard Model of elementary particles [16].

3. 3. Electromagnetic interactions

3.3.1 Electric charge as a Coriolis force in rotating frames

In certain aspects electrons behave like point-like objects, e.g. in Bhabha experiments studying the scattering of positrons by electrons [3, 4], the scattering cross section is found zero within the precision of the experiment. In the screw model it can be interpreted by the zero surface of sphere due to the rotation with the speed of light. But other properties of electrons can be explained by a finite radius, e.g. the magnetic moment. This latter property can be related to the finite R_c central radius. In the frames of the double-rotation, besides the centrifugal force, the Coriolis force [17] also appears because the second rotation will produce a motion with the speed u = r.ω in the frame of first rotation:

F_Coriolis = 2m.ωxu                                                        (20)

On the surface of the sphere at r = R_c, the peripheral speed of the second rotation is c, and this is perpendicular to the axis of other rotation, thus the Coriolis force is F_Coriolis = ±2m.ω.c. This force exerts a twist on the surface of the sphere, the sign ± describes the polarization of twist: it will be right-handed when the chirality of the double-rotation has this handedness, or left-handed in the opposite case. Since the angular momentum is ħ/2 and ω = c/R_c, the Coriolis force can be given as:

F_Coriolis = ±ħc/R²c                                               (21)

This force uniformly twists the entire 4R²_c.π surface of the sphere and generate virtual one-dimensional rotations, which we have postulated earlier as photons. The interaction mediated by the virtual photons is proportional to the total torsion force (the flux is ±4πh.c). Following the principle of QED, we assume that the electric force is mediated by virtual photons and the resulting interaction is proportional to the Coriolis flux. The electric charge in the Coulomb force:

F_Coriolis = ±e²/r² = ±α.ħ.c/r²                                            (22)

can be interpreted by the fine structure constant of Sommerfeld [18] α = e²/ħ.c = 1/137.036, which can be considered as an attenuating factor of  the Coriolis force. This attenuation factor is also a structural property of space-time similarly to the Planck constant and the speed of light. The Coulomb force is attractive if the two charged fermions have opposite chirality, and repulsive for fermions having the same chirality.

The zero charge of photons is explained by the cylindrical screw motion, since in this case the direction of propagation is parallel to the rotational axis, which produces zero Coriolis force. In the case of neutrinos the charge neutrality requires a further assumption of a superimposition of left- and right-handed screws. This assumption harmonizes with the basic principle of superposition of equivalent quantum states.

3. 3.1. Anomalous magnetic moment of electron

The theory of QED can reconstruct the anomalous magnetic moment of electrons with a very high accuracy [19]. Here we offer visualization for the major terms given by the perturbation computation of QED. Two kinds of motion of the electric charge can contribute to the magnetic moment: the orbital motion around a nucleus and the self-motion of electrons. The first contribution is proportional to the orbital quantum number L and the second to the spin S. The magnetic moment derived from the Dirac equation [14] is:

μ = μ_B(L + 2S)                                                          (23)

Here μ_B is the Bohr magneton.

According to the classical electrodynamics, the rotating charge produces magnetic moment μ = i.f/c, where the current I = e.ν, the area of circle f = r²π, thus the magnetic moment of orbital motion is:

μ_L = e.ν.r²π/c = e.ω.r²/2c                                                                                (24)

The relation between the quantum mechanical and classical orbital angular momentum: L.ħ = m.ω.r², gives the following orbital contribution:

μ_L =L. e.ħ/(2m.c) = μ_B.L                                                       (25)

The spin contribution can be computed analogously if we place the charge uniformly on the surface of the sphere at the radius r = R_c. The sphere can be decomposed into circles and the angular and magnetic moment can be obtained by integration. The question has been raised by Feynman [20], what is the origin of factor 2 in the Zeeman interaction. The answer is the difference between the one- and two-dimensional rotations. When we place the electron in magnetic field it induces a one-dimensional rotation. This rotation is superimposed on the two-dimensional rotation of the spin. It means that the spin contribution of magnetic moment is the same as obtained from the orbital motion if L = 1, but the spin is smaller by a factor two since S = ½, and thus the total contribution can be given as:

μ_S = 2μ_B.S                                                               (26)

The anomalous magnetic moment has further contributions, which are explained by the QED as coming from vacuum polarization [21].  In the one-loop approach individual photon absorptions and emissions are taken into account

μ_S = (2 + α/π)μ_B.S                                                        (27)

The theory of QED gives further terms as a power series of the fine structure constant α, when combined photon processes are taken into account. In the screw model these terms can be interpreted as the impact of Coulomb force in the stabilization of self-rotation: the centrifugal force is balanced by the sum of the strong gravitation and the Coulomb force:

2m.ω²R_c = ħ.c/R²_c + e²/(2πR²_c) = (1+α/2π)ħ.c/R²_c                                      (28)

The Coulomb force is divided by 2π since the balance extends in the whole perimeter. Eq. (28) can be rewritten:

ω.R²_c = (1+α/2π)ħ/2m                                                  (29)

By this correction the anomalous magnetic momentum of electrons can be obtained. It is not our purpose to derive the exact formula of magnetic momentum, only we want to demonstrate that the screw model is a useful visualization of the QED formalism. This interpretation also suggests that the rest mass of fermions could have a minor electromagnetic contribution beside the predominant strong gravitation. The above interpretation of the anomalous magnetic moment agrees with the results of MacGregor [22] that the Compton-sized  electron model quantitatively reproduces the spin, magnetic moment, and gyromagnetic ratio of the electron, correct to first order in α.

Though the theory of QED has great successes explaining certain properties of elementary particles, e.g. the anomalous magnetic moment of the electron, it still encounters divergence problems in the computations of self-energy [21]. The screw model may help to get rid off some of the divergences. The divergence can occur, when point like particles are assumed, since in this case the integration includes the space domain, where the radius is zero and the potential energy is infinite. In addition to the integration includes also virtual photons with infinite energy. In our screw model the point-like particles move on a finite sphere R_c and no mass and charge exist inside this sphere. Furthermore the double-rotation takes place by the de Broglie frequency, which could be the upper limit for the virtual photons. If these limitations are included in the field theories, the divergence may be avoided.

4. Conclusions

In order to rationalize the arrow model of Feynman [1] where he offers visual representation for the complicated calculations in the theory of QED, we have developed a screw model for particles. Both the photons and fermions are represented by rotation of space points with the peripheral speed of light either on the surface of a cylinder or on a sphere, respectively. The screw model is based on rotational frames, which leads to a non-Euclidean space, in which the curvature determines the force of gravitation. The function of curvature is designed to reproduce the Newton formula of gravitation and this formula is also suitable to determine the curvature, even when the rotation takes place at the speed of light. In this case the potential energy is found to be equal to –mc², which indicates that the rotation with the speed of light can produce the mass for the particles. The respective force termed as strong gravitation supplies the centripetal force balancing the centrifugal force of rotating object. The self-rotation of particles can also explain the origin of the spin, the rest energy and the magnetic moment.

The screw model suggests a new interpretation for the Planck constant defining it as a force constant of the strong gravitation. The quantum nature of micro-world can be considered as a consequence of the enormous jump of force by crossing the surface of the rotating sphere. The strong gravitation exceeds the standard gravitation by 32-42 order of magnitudes. The electric charge is interpreted as an attenuated Coriolis force of the self-motion. The sign of the charge and the particle-antiparticle duality are assigned to the chirality of the double-rotation. The parity violation in the beta decay of neutrons is explained by the incomplete character of usual reflection. If the reflection is extended to the space of self-rotations, which is an equivalent symmetry operation with the charge conjugation, the parity is no more violated. The anomalous magnetic moment of electrons can also be interpreted by the screw model. The divergence problems in the self-energy computations could be resolved if the particles are represented by rotations on a sphere with finite size and frequency. The photon mediated electromagnetic interaction is visualized by emission and absorption of the cylindrical rotations, which are produced by the spherical rotations of fermions.

 Further subjects of the blog, see: "Paradigmaváltás a fizikában"

References

[1]        R P Feynman QED, The strange theory of light and matter (Penguin books, Princeton University Press) (1985)

[2]        A Zee Quantum Field Theory in a Nutshell,. (Princeton University Press)  2nd Ed. (2010)

[3]        H J Bhabha  Proc. Roy. Soc. A154 195 (1936)

[4]        A Arbuzov, M Bigi, H Burkhardt et al. Phys. Lett. B. 383 238 (1996)

[5]        R P Feynman Quantum Electrodynamics (Westview Press) New Ed. (1998)

[6]        A O Barut and Nino Zanghi Phys. Rev. Lett. 52, 2009 (1984)

[7]        M Rivas J. Phys. A: Math. General 36,  4703 (2003)

[8]        S Ghosh, A Choudhury and J K Sarma Indian J. Phys. 86 481 (2012)

[9]        A Einstein Annalen der Physik 49 (1916),  archived from the original on 2006-08-29, retrieved 2006-09-03

[10]    K Schwarzschild Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften 7 189 (1916)

[11]    P W Higgs Phys. Rev. Lett. 13 508 (1964)

[12]    P A M Dirac Proc. Roy. Soc. A117 610 (1928)

[13]    T D Lee and C N Yang Phys. Rev. 104 822 (1956)

[14]    L Foldy and S A Wouthuysen Phys. Rev. 78 29 (1950)    

[15]    H Bethe Ann.  Physik 395 133 (1929)

[16]    L K Gordon Modern Elementary Particle Physics (Perseus Books) (1987)

[17]    G Joos and I M Freeman Theoretical Physics (New York, Courier Dover Publication) (1986)

[18]    C M  Sommerfeld Phys. Rev. 107 328 (1957)

[19]    G Gabrielse, D Hanneke, T. Kinoshita, M Nio and B Odom Phys. Rev. Lett. 97 030802 (2006)

[20]    R P Feynman, R B Leighton and M Sands The Feynman Lectures on Physics (Addison-Wesley Publishing, Massachusetts) Vol. 2, (1964)

[21]    R P Feynman Phys. Rev. 74 1430 (1948)

[22]    M H MacGregor Foundations  Phys. Lett. 2 577 (1989)